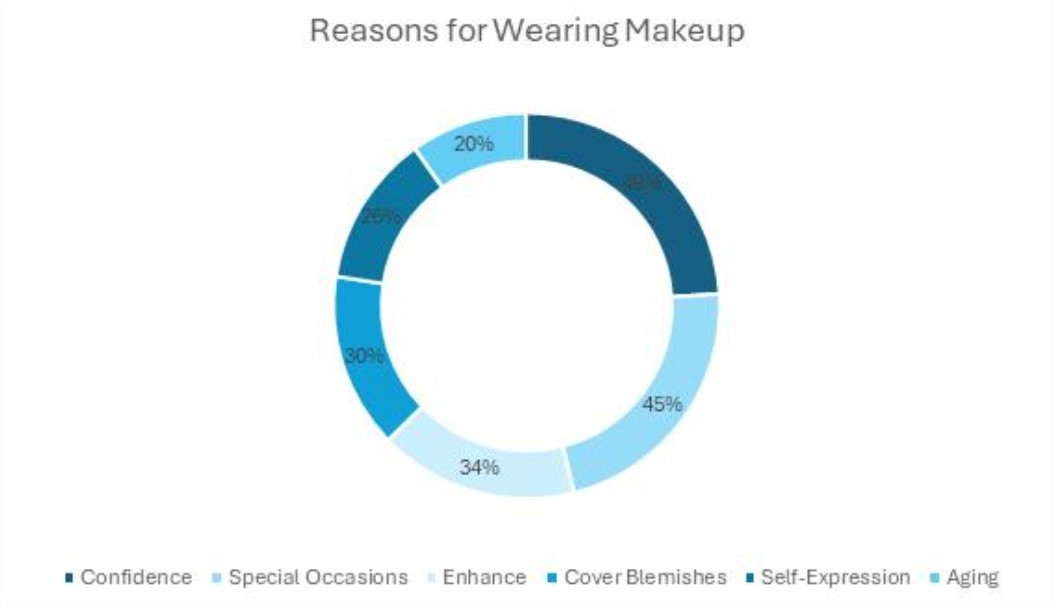
People who do not believe math and physics can be fun astound me.
A few years back, I was watching an old western movie with my brother. Just about every character was being thrown through saloon windows at a disturbing rate; however, this seems surprisingly common for this genre.
Suddenly, my brother announced that if he had to go back in time, he would choose to go to the “wild west” and be a window maker because he would seemingly have a never-ending demand for saloon windows.
Being forced to answer the same question, I would certainly go to ancient Rome and be a military engineer. A thousand years after Rome, Leonardo DaVinci would take the same profession and if it is good enough for him, it is good enough for me.
Among my works, I would beat Charles Darwin to the punch, introduce linear perspective, and “invent” the trebuchet.
Implementation is the point that math and physics get fun.
For those who do not know, a trebuchet is a powerful siege weapon designed to raze any structure to the ground. It operates using a simple lever on a fulcrum in a similar fashion as a see-saw. A heavy counterweight produces tremendous velocity and a heavy projectile is slung great distances.
The applications for a trebuchet in the Roman world would be somewhat limited based upon the relative absence of large fortifications on scale with medieval Europe; however, applications would not be non-existent.
For an example of how a trebuchet works, let’s say a group wanted to attempt to lay siege, to pick an arbitrary location, on McFall, Mo. This is how they would do it:
After collecting the necessary resources, they would set out to build the ideal trebuchet. An elevated fulcrum is driven through a long beam. The counterweight portion consists of a heavy weight of roughly 100 times the weight of the projectile while the opposing end of the fulcrum is between three and 3.75 times longer than the counterweight portion.
A sturdy frame is required to combat mounting centrifugal forces which would tear the trebuchet apart. A productive fulcrum for a large trebuchet would be eight feet in the air and the beam’s support would be perpendicular to the base. An eight foot base would create right triangles with sides of eight and four feet long.
A necessary support would need to be included at 45 degree angles. With side A being eight feet and side B being four, the hypotenuse of the triangle, the support beam, would need to be just under nine feet.
The counterweight is manually raised in the air, upon the fulcrum, to create potential energy. Upon the releasing of a trigger, gravity pulls the counterweight down and pivots the long arm, with the projectile held within a sling, in to the air. A pin keeps the sling from releasing until a desired degree of release is achieved— with 45 degrees generally being considered an optimum degree.
With a sling properly attached to the long arm of the lever, an arm totaling in length of about 20 feet, a sufficient counterweight and assuming a lack of any non-restrictive weather conditions, this trebuchet would be capable of firing an 8-14 pound bowling ball over 300 feet in a parabolic arc. This is sufficient to cover the distance from the Student Union to McFall, Missouri’s namesake building— McFall Hall.
Neither I nor the University’s administration, housed in McFall Hall, can condone using a trebuchet to raze either of these locations. I merely wish to demonstrate what is possible and that math, physics and history can work together to create something that is a lot of fun.
Respond to Greg at